Relationships Between Fractals and other Concepts
Since fractals are related to so many things in nature, in science, and in math, it's hard to narrow down what connections to discuss. Throughout the entirety of this website, you can find connections between fractals and other topics, but here I want to highlight just a few more connections.
One connection of interest is the relationship between Pascal's triangle and Sierpinski's triangle. Pascal's triangle is an expansion of the binomial coefficient, often used when working with polynomials, discrete mathematics, as well as other things. When certain multiples are highlighted, interesting patterns appear, including that of Sierpinski's triangle. Use this applet to discover patterns of fractals within Pascal's triangle.
Fractals also have a connection with infinity and zero. The Koch Snowflake technically has an infinite perimeter yet has a finite area. Additionally, the Menger Sponge has infinite surface area yet zero volume. This is mind-boggling, but if you consider the construction of the sponge, it makes sense.
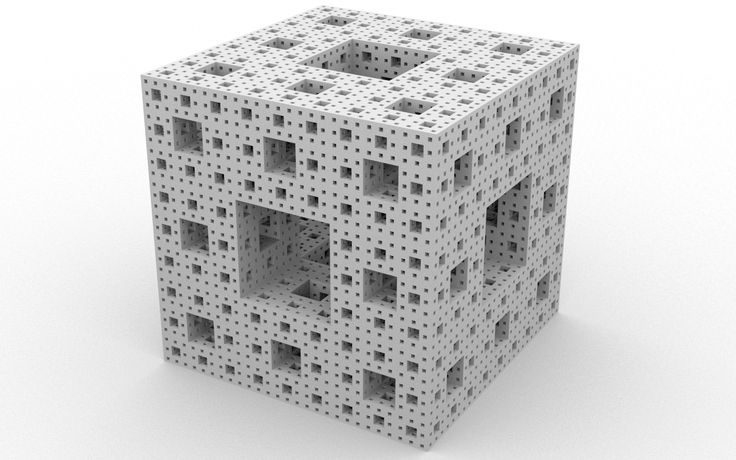 Menger Sponge -- Image Source: CurseForge
Menger Sponge -- Image Source: CurseForge
As briefly mentioned in the historical introduction, fractals play a large role in being able to describe chaos in the dynamic systems here on earth. Chaos is generally perceived as an unorderly thing by definition, but many mathematicians and scientists who have studied chaos recognize the connection to fractal geometry.