The History of Perfect Numbers
The perfect number 6 was associated with marriage, health and beauty in the time of the Pythagoreans. Because 6's proper divisors add up to neither to large or too small with regard to the number itself, Nicomachus acclaimed "that perfect numbers strike a harmony between the extremes of excess and deficiency" (Voight). Hrotsvit, one of the first female German poets, stated in her play Sapientia, "We should not leave unmentioned the principal numbers . . . those which are called "perfect numbers." These have parts which are neither larger nor smaller than the number itself, such as the number six, whose parts, three, two, and one, add up to exactly the same sum as the number itself. For the same reason twenty-eight, four hundred ninety-six, and eight thousand one hundred twenty-eight are called perfect numbers" (Voight).
Although all these individuals throughout history have identified ties between the empirical world and the perfect numbers, the great mathematician Euclid, who is believed to live in Alexandria, Egypt around 300 BC, was instrumental in the study of perfect numbers. Euclid was the first to really categorize perfect numbers.
The first four perfect numbers are 6, 28, 496, and 8128. Ancients observed that these perfect numbers end in an alternating pattern of 6's and 8's. On top of this, 6 is the only perfect number between 1 and 10, 28, the only one between 10 and 100, 496 is the only one between 100 and 1,000 and 8,128 is the only perfect number between 1,000 and 10,000. Both of these patterns were assumed to hold for the subsequent perfect numbers, but unfortunately, these patterns fail quickly. The fifth perfect number is 33,550,336, which follows the alternating pattern of 6's and 8's, but fails to fall between 10,000 and 100,000 by a factor of 1000. Sadly, the next perfect number breaks the first pattern being 8,589,869,056 and ending in a 6 instead of an 8. Although this pattern does not continue, all perfect numbers discovered end in either a 6 or an 8.
Euclid noticed with the first four perfect numbers (the ones that were known at the time) that all of them were produced with the formula (2n-1)(2n-1). He then went on to prove his formula, now a theorem, that every time (2n-1) is prime an even perfect number is produced. "Euclid's proof got the theory of perfect numbers off to a roaring start, but the nearsightedness of other mathematicians made progress slow. Many fine minds thought they saw patterns in the numbers where none existed. If they looked a little further, they would have seen that the patterns were illusory" (Hoffman), like an alternating end pattern of 6's and 8's, or the spot they occupy between 0 and 10, 10 and 100, etc.
The first four perfect numbers were known to the ancient Greeks, although who discovered them is unclear. After the first four, the fifth perfect number was not discovered until the 15th century (1456) by whom we don't know. The sixth and seventh perfect numbers were discovered in 1588 by an Italian mathematician by the name of Pietro Cataldi. The 8th perfect number was discovered by Leonard Euler in 1772, and the 9th and 12th discovered in the late 1800's by two different individuals. From 1911 to 2017, forty out of the fifty perfect numbers were discovered, the last consisting of more than 46 million digits. The table below shows each perfect number with whom they were discovered by in what year, and the number of digits each contain:

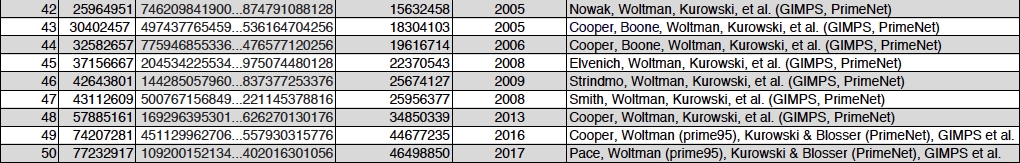
(Wikipedia, Caldwell)
All perfect numbers discovered are even, and it has been proven with the Odd Perfect Number Conjecture that no odd perfect numbers exist.
Another type of number that is closely tied with perfect numbers are those called Mersenne primes. "Numbers generated by the expression (2n-1) are now known as Mersenne Numbers, after a seventh-century Parisian monk, Marin Mersenne, who took time out from his monastic duties for number theory" (Hoffman).
The Mersenne Primes are a subset of the Mersenne Numbers. So, a Mersenne Number is a Mersenne Prime if n is a prime integer and (2n-1) is also a prime integer. With every discovery of a Mersenne Prime, there is a discovery of a perfect number as well. Typically, the Mersenne Prime precedes the discovery of the perfect number. The formula that has been developed to produce perfect numbers can be proved using this unique relationship.
See Explanation of the Mathematics for more details of this proof.