Marissa's Class

As described before the most basic definition of volume is multiplying length, width, and height to determine in squared unites the number of cubic units can fit inside the object. This works simply with cubes and cuboids because we can easily visualize and measure each side length as shown below in figure 1. However, as the object becomes more complicated, it becomes increasingly difficult to visualize putting blocks inside the object.

To find the formulas for volume of more complex three-dimensional shapes, the idea is used of breaking down the shape into small rectangles of which the volume can be calculated. For example, a rectangular pyramid may be used (see video below)

Then the sum of the volumes of each rectangle can be used to approximate the volume of the pyramid. You will notice that as the number of slices increases, the approximation of the volume increases. Adding together each of these slices and calculating the summation as n goes to infinity gives us the formula for a rectangular pyramid. The same can be done for similar shapes such as cones. They will instead have a circular base. Following a similar process, the formula for the volume can be found. This process in essence is integration to find the volume.

Other irregular shapes may require double integration to find the volume. This would be the case for objects whose base is not simple shapes of which are not easy to calculate their volume. Some examples are shown below (see figure 3).

Double integration is a powerful mathematical technique employed to find the volume of complex three-dimensional shapes. It extends the principles of calculus to solve problems involving intricate geometries. To apply double integration for volume determination, one considers the shape as a stack of infinitesimally thin cross-sectional slices, each of which can be represented by a two-dimensional area. By integrating these areas over the entire span of the shape, both horizontally and vertically, we effectively sum up the volumes of these infinitesimal slices, thus yielding the total volume of the complex shape. This method is particularly valuable when dealing with irregular and non-uniform shapes, as it allows for a precise and versatile approach to calculating their volume, making it an indispensable tool in fields like engineering, physics, and computer-aided design.

Together we will consider this example and the use of double integration. First consider the shape created by \$f:z=3-x^2-y^2$\and \$g:z=2x^2+2y^2$\ in the volume applet.

Volume applet

Instructions

Use the Slider to slice the object

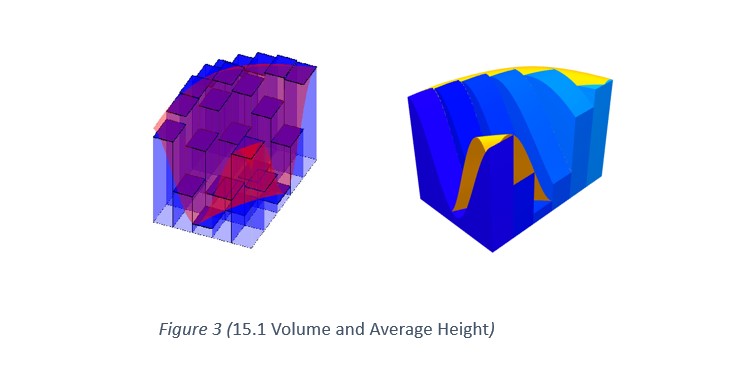
Move point B to move the line displaying the height of the object at that point.

To find the volume of this shape we must integrate $ f-g $ over an area $ D $ and first, we must find where these two objects intersect to find the area $ D $. We do so by setting the equations equal to each other.

$ f(x,y)=g(x,y) \\ 3-x^2-y^2=2x^2+2y^2 \\ 3=3x^2+3y^2 \\ 1=x^2+y^2 $

We notice that in this case, the curve where the objects intersect is the unit circle. This means that we will integrate over the region $x^2+y^2≤1$. This gives us the area of the object’s base or the length times width of the object. To find the height of the object we must subtract f-g.

$ f-g\\ 3-x^2-y^2-2x^2+2y^2\\ 3-3x^2-3y^2\\ $

Now we will use the idea of integration based on the idea of splitting this shape into infinitely many slices to calculate the volume of the object. We will integrate the 1 over region D.

$ \iint (3 - 3x^2 - 3y^2) \, dy \, dx\\ \iint\limits_{-\sqrt{1-x^2}}^{\sqrt{1-x^2}} (3 - 3x^2 - 3y^2) \, dy \, dx\\ \int_{-1}^{1} (6\sqrt{1-x^2} - 6x^2 \sqrt{1-x^2} - 2(1-x^2)^{3/2}) \, dx\\ \int_{-\pi/2}^{\pi/2} (6\sqrt{1-\sin^2(\theta)} - 6\sin^2(\theta)\sqrt{1-\sin^2(\theta)} - 2(1-\sin^2(\theta))^{3/2}) \cos(\theta) \, d\theta\\ \int_{-\pi/2}^{\pi/2} (6\cos(\theta) - 6\sin^2(\theta)\cos(\theta) - 2\cos^3(\theta)) \cos(\theta) \, d\theta \\ \int_{-\pi/2}^{\pi/2} 4\cos^4(\theta) \, d\theta\\ \int_{-\pi/2}^{\pi/2} \left(\frac{3}{2} + 2\cos(2\theta) + \frac{1}{2}\cos(4\theta)\right) \, d\theta = \frac{3\pi}{2}\\ $

Using integration, we get 3π/2 is the volume of this object. On occasion it is sometimes helpful to consider using polar coordinates and trigonometry to make integration easier over circular based shapes. This is one of those cases. For example, using polar coordinates the integration goes as follows.

$ v = \iint (3 - 3x^2 - 3y^2) \, dy \, dx\\ = \int_{0}^{2\pi} \int_{0}^{1} (3 - 3r^2) \, r \, dr \, d\theta \\ = \int_{0}^{2\pi} \left(\int_{0}^{1} \left(\frac{3}{2}r^2 - \frac{3}{4}r^4\right) \, dr \right) \, d\theta\\ = \int_{0}^{2\pi} \left[\left(\frac{3}{4}r^3 - \frac{3}{20}r^5\right)\Big|_{r=0}^{r=1}\right] \, d\theta \\ = \int_{0}^{2\pi} \left(\frac{3}{4} - \frac{3}{20}\right) \, d\theta\\ = \int_{0}^{2\pi} \frac{3}{5} \, d\theta = \frac{3\pi}{4} $

We can see that using either method we get the same result for the area of the object.

Exploration of Mathematics