So What is Chaos In Mathematics?



While Lorenz is considered father of modern Chaos, James Yorke originally coined the termed "chaos". In fact, it was actually some time before Lorenz received due credit for his contribution. This was owing to the fact that his paper on such systems was hidden from the mathematics and physics community in an atmospheric studies journal.

So what is Chaos? A better question may be " what constitutes a chaotic system " ? Sensitivity to initial conditions is a major component of this branch of mathematics, but there are a few other criteria and characteristics that ought to be discussed.

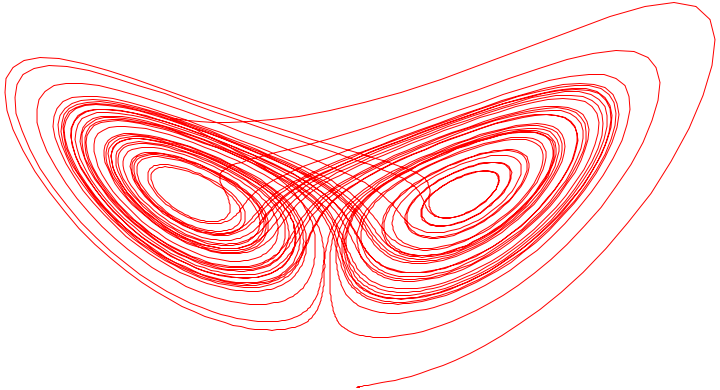
The first of these is topological mixing. Suppose we have two trajectories of the same chaotic system that differ in their initial conditions. At any point in time, the neumeric solutions could be very, very different- as was seen in the Lorenz attractor applet. However, topological mixing means that no matter how far apart these points are at one moment, we could find them arbitrarily close the next because their trajectories share the same phase space. To illustrate with a non-example, suppose a pilot takes off from an air-port with a fixed destination but a faulty compass that doesn’t point quite north. As a result, his direction may deviate by a fraction of a degree from his supposed destination. After traveling the requisite miles, he may be quite distant from his destination. Sensitivity to initial conditions? Sure. But is this an example of chaos? Not quite. Not only can the pilot determine where he is simple by knowing to what degree his trajectory is off, but that little difference in direction resulted in two very different spaces over a period of time. These solution spaces were not topological mixed.

Another defining characteristic of these dynamic systems is the dense periodic orbits they display when graphed using numerical methods for a sufficient amount of time. This has been illustrated with the Lorenz attractors in a previous page as well.

Though real world chaotic systems surround us each day, mathematically, these systems are really quite simple. Note how the Lorenz equations contained but three variables and three constants. Intuitivly, many of understand that a large number of interacting varaibles can make a system unpredictable- there are countless examples in the in the world around us. Interestingly, very simple mathematical systems can exhibit the same chaotic behavior. Additionally, they can be used to model complex real-world system and teach us about their behavior. Below is an applet modeling the behavior of a double pendulum. It illustrates how extreamly simple, deterministic systems can behave unpredicably.

Importaint to note, however, is though the behavior of these systems is certainly unpredictable, the systems are still deterministic- not random. This is another way of saything that the initial conditions of these systems of equations will completly determine all future outcomes and that there will be no randomness involved in the set of its solutions. Given the same initial condition, a system will behave exactly the same way every single time.

But again, the things about these systems is that though they are deterministic, they still lack analytical solutions and require numerical methods to evaluate. Such computer modeling techniques have given us visual representations of these systems that can provide us deeper insight into the world of chaos. Again, because no analytic solution exists for such systems, we can still only ever predict an outcome and, as our extrapolations into the future become greater, our error grows exponentially.

The seeming paradoxes don't end there either. Though chaotic, they still exhibit order- as was the case with the Lorenz attractors seen above. The trajectories orbited around what are known in the field as strange attractors. An attractor is merely a value or set of values that a system will converge to over time. The Logistic Map discussed on the next page models such attractors for certain values of r. A sysystem with a strange attractor, on the other hand, will tent to continually oscillate around a value or values but never actually reach the attractor. Such systems will never repeat but continually exhibit similar, though unpredictable, behavior. The Lorenz Attractor Applet on the previous page is an illustration of strange attractors.

Mathematically, these systems fall in the domain of non-linear dynamics and are often describe as such. Here the word "dynamic" indicates that the function is dependent on a time variable. Additionally, non-lineararity can be be understood to mean that the function can not be graphed as a simple line. The author James Gleick illustrated the chaotic nature of these systems by rephrasing nonlinearity as meaning " that the act of playing the game has a way of changing the rules ".

< Previous Next >