Proofs of Trigonometric Identities

History of Trigonometry Semester Project Introductory Page Unit Circle Relationship Application of Trigonometric Identities


In the article “History of Trigonometry-Part 3” the purpose of trigonometric identities is stated: “The study of identities was important because by establishing relationships between sums and differences, and fractions and multiples of angles, more efficient astronomical calculations could be conducted, and more accurate tables could be established” (Rogers, 2010). The most common trigonometric identities are the Pythagorean identities, the sum and difference formulas for sine and cosine, the double angle formulas, and the sum to product identities (Joyce, 1997). Understanding the development of these identities proves the importance of them in trigonometry.

Mathematicians use various techniques in mathematics to prove theorems and identities. These techniques have been applied when it came to the discovery of trigonometric identities. The proofs are rooted in the relationship of right triangles inscribed within the unit circle. In the article called “An historic-geometric development of trigonometric concepts”, figure 1 is used to illustrate the right triangles that can be created based on properties of the unit circle. In the triangle ABC the line segment AC is the radius of the unit circle, therefore it has a length of one. The trigonometric ratios of cosine and sine are constructed based on the triangle ABC where theta is the angle CAB. The definition of sine is the opposite length of theta and cosine is the adjacent length of theta.(Murphy, 1998). The tangent and secant are defined by constructing another right triangle ADF on the unit circle. We can construct the line AD from 0 to 1 on the x-axis. Then a perpendicular line is constructed from point D and the ray AC from the last triangle can be extended to intersect with the perpendicular line that goes through point D. Point F is constructed where the two lines intersect. The definition of tangent is the length of ADF opposite of theta and secant is the hypothenuse of ADF. Now cosecant and cotangent are defined by constructing a final right triangle AGH on the unit circle. The line segment AG is constructed on the y-axis with a length of 1. Then a perpendicular line is constructed to the line segment AG and goes through point G. The ray AC can be extended to intersect with the perpendicular line and point H is the place where these two lines intersect. The triangle AGH is then constructed. These constructions are described by the figure on the right (Murphy, 1998). The applet trigonometric functions show a visible representation of these trigonometric relationships described above.

Pythagorean Trigonometric Identities

The Pythagorean trigonometic identiies were developed from right triangles constructed in the unit circle. Here is the construction and proof for the cosine and sine pythagorean identity. The right triangle ABC follows the following construction. A is the center of the unit circle with point C on the circle. The line segment AC is constructed. Then a perpendicular line is contructed to the x-axis and the point C. Point B is where the perpendicular line and the x-axis intersect. The line segments AB and CB are then constructed, which creates the triangle ABC. This construction is represented in figure 1. This construction is used to prove that \( \cos^2{ \theta } + \sin^2{ \theta } = 1 \). Since ABC is in insrbed in the unit circle and C is on the unit circle then AC = 1. We will show that \( AC^2 = AB^2 + BC^2 \) is equal to \( 1 = cos^2( \alpha ) + sin^2( \alpha ) \). Since ABC was constructed as a right triangle then by trigonometry \( AB = cos( \alpha ) \) and \( CB = sin( \alpha ) \). We can substitute for AB and CB as \( AC^2 = cos^2( \alpha ) + sin^2( \alpha ) \). We used the Pythagorean theorem to find AC. Now we have defined AC = 1 so we can substitute that in to our equation to get \( 1 = cos^2( \alpha ) + sin^2( \alpha ) \) Q.E.D. (Side note Q.E.D means end of proof)(Murphy, 1998).

The construction and proof for the tangent and secant pythaogrean identity is similar to the proof mentioned above. Let A be the center of the unit circle and D be the point (1,0) on the unit circle. A line segment can then be constructed to form AD. Then construct a line perpendicular to AD and goes through point D. Another line is constructed to go through A and intersect with the perpendicular line. Point E is the place of intersection. The right triangle ADE is constructed and the figure below shows this construction. With this construction we will prove that \( AE^2 = AD^2 + DE^2 \) is equal to \( sec^2( \alpha ) = 1 + tan^2( \alpha ) \). Now since the right triangel ADE is inscrbed the unit circle by construction then AD = 1. Then by the definition of tangent \(DE = tan( \alpha ) \) and by the definition of secant \(AE = sec( \alpha ) \). Now we since ADE is a right triangle we can use the pythagorean theorem, so \( AE^2 = AD^2 + DE^2 \) (1). Now substitute AE, AD, and DE as defined earlier in equation 1. We get \( sec^2 ( \alpha ) = 1 + tan^2( \alpha ) \)(Murphy, 1998).

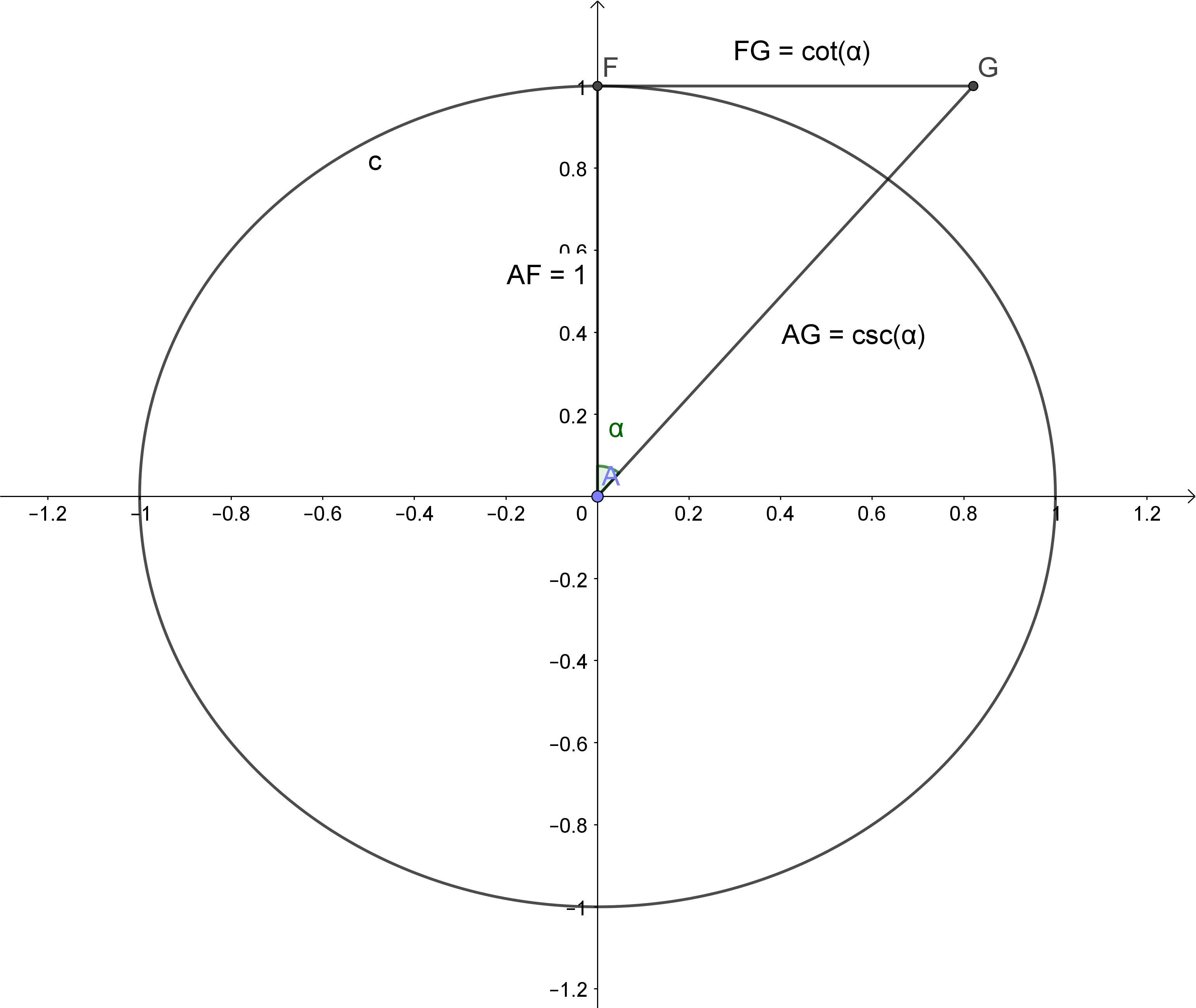
The last Pythoagorean Identity is the cotangent and cosecant identity and its construction is very similar to the other pythagorean identities. Let A be the center of the unit circle with point F at (0,1) on the unit circle. The line segment AF is constructed. Create a line that is perpendicular to AF and goes through the point F and create a line that goes through point A and intersect with the perpendicular line. Point G is the place where the line and the perpendicular line intersect. Construct the right triangle AFG. Based on our construction we will show that \( AG^2 = FA^2 + GF^2 \) is equal to \( csc^2( \alpha ) = 1 + cot^2(\alpha) \). By construction AFG is a right triangle on the unit circle then AF = 1. \( cot( \alpha ) = FG \) by definition and \( csc( \alpha ) = AG \) also by definition. We are given that \( AG^2 = AF^2 + FG^2 \) (1). Now we can substitute AG, AF, and FG with its definitions, so we get \( csc^2( \alpha ) = 1 + cot^2( \alpha ) \)(Murphy, 1998).



Next