
The Pythagorean Theorem is one of the most recognizable theorems in mathematics. This Theorem gives us a relationship among the sides of a right triangle: the sum of the legs squared is equal to the hypotenuse squared.
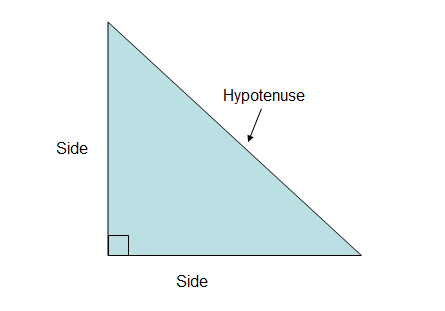
A right triangle is a special type of triangle. It is a triangle with one angle equaling 90 degrees. Now because the measure of the interior angles of a triangle is equal to 180 degrees; we also know that the remaining two angles will sum to be 90 degrees as well. A right triangle has two legs-- which are the shorter two sides of the triangle, and a hypotenuse-- which is the longest side of a right triangle, and is located directly across from the right angle. The altitude of a right triangle is equal to a leg in the right triangle.
Pythagoras, a Greek mathematician, is credited with the discovery of the Pythagorean Theorem although we know that the Babylonians understood the theorem much earlier. The Pythagorean Theorem can be proven in a variety of ways using: similar triangles, rectangles, and squares.
Here is an example of one type of proof for the Pythagorean Theorem .
This applet,Pythagorean Puzzles , allows you discover the Pythagorean Theorem by moving pieces of squares and triagnles into a square.
This original applet, Pythagorean Theorem allows you to conjecture about the relationships among area of shapes attached to the sides of a right triangle. By using the area of known shapes you can prove that the relationship of the areas follows the Pythagorean Theorem. Thus you can conclude that it is true. Here is an activity plan and a task sheet to go along with the applet.